How to Find Sample Variance in Excel?

Do you need to know how to calculate sample variance in Excel? Calculating sample variance in Excel is a simple process that can be done with a few clicks of the mouse. In this article, you will learn how to find sample variance in Excel and the steps involved in doing so. By the end of this article, you will be able to apply sample variance calculations to your data sets. So, let’s get started!

Understanding Sample Variance

Sample variance is a measure of the spread of a data set. It is calculated by taking the square root of the average of the squared differences from the mean. This helps to measure how far each value in the data set is from the mean. Sample variance is used to determine how volatile a data set is, or how much the values in the data set vary from the mean.

Sample variance is an important tool in statistics and data analysis. It can be used to make predictions about future data sets, or to compare different data sets. It can also be used to determine the accuracy of a data set, or to identify outliers.

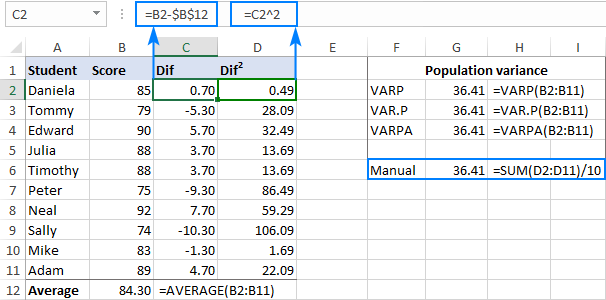
Calculating Sample Variance Manually

The formula for calculating sample variance manually is as follows: first, calculate the mean of the data set. Then, subtract the mean from each value in the data set. Square the differences, and sum them up. Finally, divide the result by the number of values in the data set minus one. The result is the sample variance.

To calculate sample variance manually, it is important to understand the concepts of mean and variance. It is also important to have an understanding of basic arithmetic and algebra.

Calculating Sample Variance in Excel

Calculating sample variance in Excel is easy and straightforward. Excel has a built-in function for calculating sample variance. This function is called VAR.S and can be used to calculate sample variance for a given range of cells.

To calculate sample variance in Excel, select the range of cells that contains the data. Then, click the Formulas tab, and select the VAR.S function. Enter the range of cells in the function, and press Enter. The result is the sample variance.

Interpreting the Results

Interpreting the results of the sample variance calculation can be difficult. Generally, a lower sample variance indicates that the data is more consistent, while a higher sample variance indicates that the data is more volatile.

It is important to keep in mind that sample variance is only one measure of the spread of a data set. Other measures, such as standard deviation, can be used to get a fuller picture of the data set.

Using Sample Variance for Predictions

Sample variance can be used to make predictions about future data sets. For example, if a data set has a high sample variance, it may be more volatile than a data set with a lower sample variance. This can be used to predict how future data sets may behave.

Similarly, sample variance can be used to compare different data sets. Data sets with a higher sample variance will tend to have more extreme values, while data sets with a lower sample variance will tend to have more consistent values.

Conclusion

Sample variance is a powerful tool for understanding the spread of a data set. It can be used to make predictions about future data sets and to compare different data sets. It can also be used to identify outliers. Calculating sample variance in Excel is easy and straightforward, and can be done using the built-in VAR.S function.

Top 6 Frequently Asked Questions

What is Sample Variance?

Sample variance is a measure of the spread of a given set of numerical data values. It is calculated as the average of the squared differences from the mean. The sample variance is an estimate of the population variance and is calculated by summing the squared differences between each data value and the population mean, then dividing the sum by the sample size minus one.

What is the Formula for Calculating Sample Variance?

The formula for calculating the sample variance is:

Variance = / (n – 1)

Where:

Σ(x – μ)2 is the sum of the squared differences between each data value and the population mean.

n is the sample size.

μ is the population mean.

How to Calculate Sample Variance in Excel?

Sample variance can be calculated in Excel using the VAR.S function. This function takes a range of values as an argument and returns the sample variance of the values. To calculate the sample variance, enter the following formula into a cell in Excel:

=VAR.S(range)

Where range is a range of cells containing the data values.

What are the Limitations of Calculating Sample Variance in Excel?

The VAR.S function in Excel can only calculate the sample variance of a dataset of up to 255 values. If the dataset is larger than 255 values, then the sample variance must be calculated manually. Additionally, the VAR.S function only works with numerical data values and cannot be used to calculate the sample variance of text or logical values.

What is the Difference between Sample Variance and Population Variance?

The main difference between sample variance and population variance is that the sample variance is an estimate of the population variance. The sample variance is calculated using the sample data, whereas the population variance is calculated using the entire population. Additionally, the sample variance is calculated by dividing the sum of the squared differences by the sample size minus one, whereas the population variance is calculated by dividing the sum of the squared differences by the population size.

What is the Use of Sample Variance?

Sample variance is used to measure the spread of a given set of numerical data values. It is a useful statistic for researchers who are looking to understand how much variation exists in a dataset. It can also be used to compare different datasets and determine if they have similar distributions. Additionally, it can be used to identify outliers in a dataset and to determine if a dataset is normally distributed.

Once you have mastered the steps of how to find sample variance in Excel, you will be able to quickly and easily analyze any given data set. With the ability to quickly and accurately analyze your data, you will be able to make informed decisions on how to best utilize the information provided and make the most of your data analysis. Knowing how to use Excel to find sample variance is an invaluable skill for any data analyst and Excel user.